We may not have the course you’re looking for. If you enquire or give us a call on +44 1344 203 999 and speak to our training experts, we may still be able to help with your training requirements.
We ensure quality, budget-alignment, and timely delivery by our expert instructors.

Understanding the Area of a Square might seem straightforward, but it’s a crucial building block for many geometric calculations and practical applications. Whether you’re designing floor plans or creating art, knowing how to calculate the Area of a Square is essential. In this blog, we’ll delve into the concept of area, break down the specific formula for a square, and walk through examples to help you master this fundamental skill.
Join us as we explore the fascinating world of square areas and equip you with the knowledge to tackle any square you encounter with confidence!
Table of Contents
1) What is Area?
2) The Formula for the Area of a Square
3) How to Calculate the Area of a Square
4) Examples of Calculating the Area of a Square
5) Common Core State Standards for Area
6) Sample Problems for the Area of a Square
7) Conclusion
What is Area?
Area is a fundamental geometric concept that measures the space within a two-dimensional shape's boundaries. Measured in square units (e.g., m², cm², in²), the area plays a crucial role in both practical and theoretical contexts. For instance, understanding the area helps determine how much space is available for furniture or how much paint is needed for a wall.
The area is calculated using specific formulas for different shapes, with the square—featuring four equal sides—being among the simplest. Similarly, the concept of area extends to more complex shapes, such as circles, where calculating the Area of Quadrant involves understanding a section of the circle. Likewise, the Area of Rhombus is determined using its diagonals, making it a crucial concept in geometry. Mastering these concepts is valuable for both academic and real-world applications.
Formula for the Area of a Square
The formula for calculating the Area of a Square is simple yet powerful:

or more commonly written as:
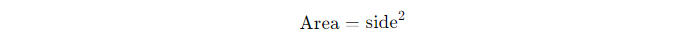
In this formula, "side" refers to the length of one of the square’s sides. As all sides of a square are equal, you only need to know the length of one side to calculate the entire area. Similarly, understanding the Area of Trapezium follows a specific formula that allows you to calculate the area based on the lengths of the parallel sides and height. This formula is foundational in geometry, including calculating the Volume of a Square, and can be applied in various contexts, from academics to real-world problem-solving.
How to Calculate the Area of a Square?
Calculating the Area of a Square is a straightforward process using the formula mentioned above. Here’s how you can do it step by step:
Measure the Length of a Side
Begin by measuring one side of the square. Ensure the measurement is in the same units you wish to express the area (e.g., metres, centimetres).
Apply the Formula
Once you have the length of the side, plug it into the formula:

Calculate the Result
Multiply the side length by itself to find the area. The result will give you the area in square units.
For example, if one side of the square is 5 metres, the area calculation would be:

Take charge of your future! Join our Career Development Course today and unlock the skills and strategies to advance your professional journey.
Examples of Calculating the Area of a Square
To better understand the concept, let’s explore a few examples of how to calculate the Area of a Square using different methods.
Using Unit Squares and Multiplying the Sides
Example 1: Using Unit Squares
Imagine a square with a side length of 4 centimetres. If you were to fill the square with unit squares (each 1 cm by 1 cm), you would count 16 unit squares inside the square. Therefore, the area of the square is 16 square centimetres.
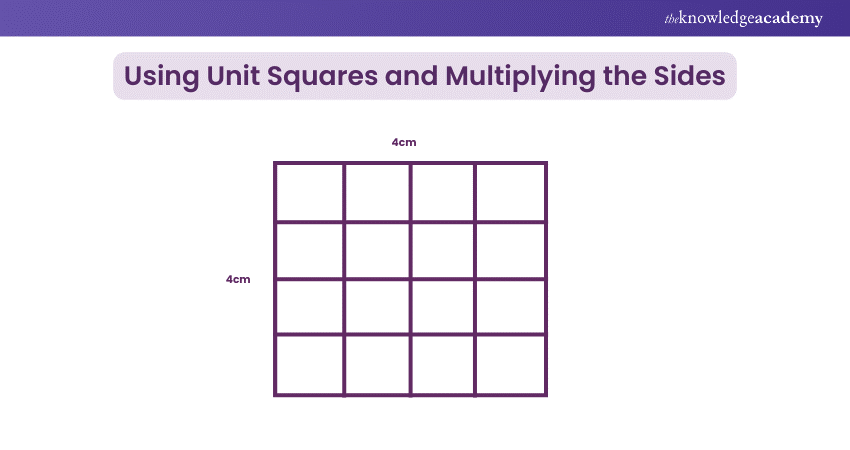
Using Unit Squares to Determine the Area
In this method, you visualise the square as made up of smaller squares (unit squares). If each side of the square is three units long, you can arrange three rows of 3 unit squares each, resulting in 9 unit squares filling the square. Thus, the area is 9 square units.

Multiplying the Sides to Find the Area
Example 2: Multiplying the Sides
Consider a square with a side length of 7 metres. Using the formula:
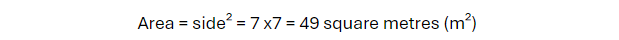
This method is quicker and more efficient for finding the area, especially when dealing with larger numbers.
Common Core State Standards for Area
The Common Core State Standards (CCSS) highlight the importance of understanding areas from an early age. Starting in 3rd grade, students learn to measure area by counting unit squares (e.g., square cm, square m, square in, square ft). As they advance, they apply these calculations to various shapes, including squares, deepening their grasp of geometry and spatial reasoning.
The standards aim to equip students with the ability to calculate areas and comprehend their practical uses, such as tiling floors, measuring land, or designing layouts. Mastering area calculations, particularly the area of squares, is essential for developing a solid mathematical foundation.
Sharpen your skills. Train to Become a Top Engineer. Join our Engineering Skills Training now!
Sample Problems for the Area of a Square
To solidify your understanding, here are some sample problems to practice calculating the Area of a Square:
Problem 1:
A square garden has sides of 10 metres. Calculate the area of the garden.
Solution:
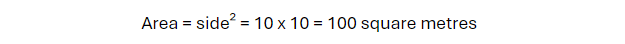
Problem 2:
A square tile has sides of 15 centimetres. What is the area of one tile?
Solution:
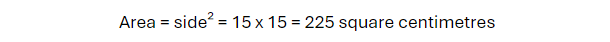
Problem 3:
If a square field has an area of 64 square metres, what is the length of one side?
Solution:
To find the side length, take the square root of the area:
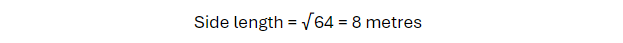
Conclusion
Mastering the Fundamentals of the Area of a Square is more than just a mathematical exercise—it’s a skill with wide-ranging applications in everyday life. From architectural designs to artistic creations, understanding this fundamental concept empowers you to tackle various projects precisely and confidently. By grasping the formula and practising with examples, you can seamlessly integrate this knowledge into your toolkit. So, the next time you encounter a square, you’ll be ready to calculate its area with ease and accuracy.
Take charge of your future! Join our Career Development Course today and unlock the skills and strategies to advance your professional journey.
Frequently Asked Questions
Can the Area of a Square be calculated using any side?

Yes, since all sides of a square are equal, you can use the length of any side to calculate the area using the formula Area= Side².
What units are used to express the Area of a Square?

The Area of a Square is expressed in square units, such as square metres (m²), square centimetres (cm²), or square inches (in²), depending on the units used to measure the side lengths.
What are the Other Resources and Offers Provided by The Knowledge Academy?

The Knowledge Academy takes global learning to new heights, offering over 3,000 online courses across 490+ locations in 190+ countries. This expansive reach ensures accessibility and convenience for learners worldwide.
Alongside our diverse Online Course Catalogue, encompassing 19 major categories, we go the extra mile by providing a plethora of free educational Online Resources like News updates, Blogs, videos, webinars, and interview questions. Tailoring learning experiences further, professionals can maximise value with customisable Course Bundles of TKA.
What is The Knowledge Pass, and How Does it Work?

The Knowledge Academy’s Knowledge Pass, a prepaid voucher, adds another layer of flexibility, allowing course bookings over a 12-month period. Join us on a journey where education knows no bounds.
What are the Related Courses and Blogs Provided by The Knowledge Academy?

The Knowledge Academy offers various Personal Development Courses, including Engineering Skills Training, Time Management Training and Active Listening Training. These courses cater to different skill levels and provide comprehensive insights into the Area of a Circle.
Our Business Skills blogs cover a range of topics related to Personal Development, offering valuable resources, best practices, and industry insights. Whether you are a beginner or looking to advance your Engineering Skills, The Knowledge Academy's diverse courses and informative blogs have got you covered.
Upcoming Business Skills Resources Batches & Dates
Date
 Engineering Skills Training
Engineering Skills Training
Fri 6th Jun 2025
Fri 29th Aug 2025
Fri 24th Oct 2025
Fri 26th Dec 2025
Fri 9th Jan 2026
Fri 6th Mar 2026
Fri 1st May 2026
Fri 4th Dec 2026






 Top Rated Course
Top Rated Course



 If you wish to make any changes to your course, please
If you wish to make any changes to your course, please


