Top Rated Course
We may not have the course you’re looking for. If you enquire or give us a call on +44 1344 203 999 and speak to our training experts, we may still be able to help with your training requirements.
Area of a Circle: A Complete Guide
Sienna Roberts 03 April 2025The Area of a Circle is the space enclosed within its boundary, calculated using the formula A = πr², where r is the radius. This fundamental geometric concept is essential in mathematics and real-world applications. In this blog, we explore its derivation, practical uses such as pizzas, fabrics and step-by-step calculation examples.
Training Outcomes Within Your Budget!
We ensure quality, budget-alignment, and timely delivery by our expert instructors.
Table of Contents
Ever stared at a delicious pizza, wondering exactly how much cheesy goodness you're about to devour? Or maybe you're designing a dream pool and need to calculate the perfect surface area. The answer to these circular questions lies in a fundamental concept: the Area of a Circle. This seemingly simple shape holds a powerful secret – its area can be precisely measured using a clever formula.
This blog cuts through the confusion and provides a complete guide to the Area of a Circle. We'll break down the formula in a way that makes sense, explore real-world examples, and show you how to solve any circular area problem with ease. So, ditch the guesswork and become a master of the circle – your pizza (and pool!) will thank you!
Table of Contents
1) What is the Area of a Circle?
2) Steps to Calculate the Area of a Circle
3) Area of a Circle Using Circumference
4) Examples of Calculating the Area of a Circle
5) Differences Between the Area and Circumference of a Circle
6) What is the Perimeter of a Circle?
7) What is the Surface Area of a Circle?
8) Conclusion
What is the Area of a Circle?
The area of a circle refers to the amount of space or region that the circle occupies. It can also be determined by calculating the total number of square units that fit inside the circle. The area covered by one full rotation of the circle's radius on a 2-D plane is known as the area of the circle. Similarly, when extending this concept to three-dimensional shapes, the area of a cylinder involves a similar principle, where the base is a circle. The area of both shapes is typically measured in square units, such as square meters (m²), while the volume of a Cone is typically measured in cubic units.
a) π (Pi) = 22/7 or 3.14, a mathematical constant presenting the ratio of a circle's circumference to its diameter.
b) r = radius of the circle (distance from the center to the boundary).
c) d = diameter of the circle (twice the radius).
This formula helps quantify the exact space within the circle's perimeter.
Steps to Calculate the Area of a Circle
Here are the basic steps to determine the area of a circle:
1) Identify the radius of the circle
2) Write the formula for the area of a circle
3) Substitute the radius value with the number
4) Calculate the area
The process of calculating the area of a circle is explored in detail below
Area of a Circle Using Circumference
The circumference of a circle can be used to calculate its area directly. The formula for the area of a circle in terms of its circumference is:
To find the area from the circumference, follow these steps:
1) Use circumference to determine the radius.
2) Calculate the area using the radius.
However, with this formula, you can bypass finding the radius and directly compute the area from the given circumference. Similarly, understanding the Area of Trapezium or Area of Rhombus can help simplify geometric calculations, ensuring accurate results without unnecessary steps. This method simplifies the process and ensures accurate results.
How to Calculate the Area Using the Circumference?
To calculate the area using the circumference, you first solve for the radius and then use the radius in the area formula. Here's the step-by-step process:
Thus, the Area of a Circle can also be expressed as
This is applicable when the circumference is known.
Examples of Calculating the Area of a Circle
To illustrate these concepts, let's go through various examples of calculating the Area of a Circle using different given parameters.
Calculating the Area of a Circle with the Radius (Answer in Terms of π)
If the radius of a circle is 5 units, the area is calculated as:
Calculating the Area of a Circle with the Diameter
If the diameter of a circle is 10 units, the radius is half the diameter.
The area is 25π square units.
Calculating the Area of a Circle with the Circumference
If the circumference of a circle is 20π units, find the area.
Find the Radius from the Circumference:
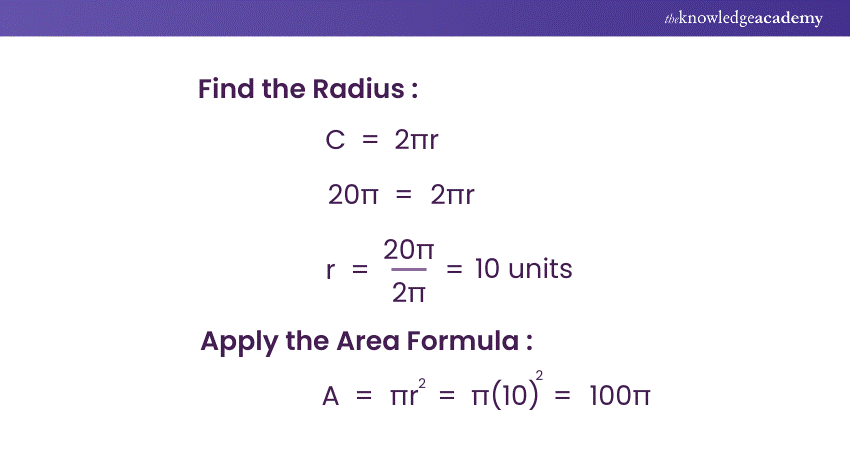
The area is 100π square units.
Unlock your potential! Register for our Personal Development Courses today to enhance your skills, boost your confidence, and realise your goals
Calculating the Area of a Semi-Circle with the Diameter
A semi-circle is half of a circle. The area of a semi-circle is half the area of a full circle with the same radius.
If the diameter of a semi-circle is 14 units, the radius is half the diameter.
Find the Radius:
The area of the semi-circle is 24.5π square units.
Calculating the Area of a Circle with the Radius
If the radius of a circle is 4 units, the area is calculated as:
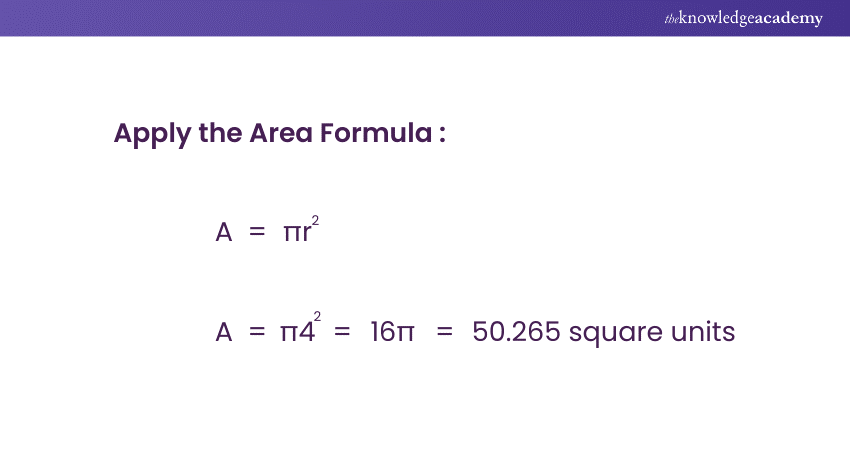
The area is 50.625 square units.
Calculating the Area of a Circle with the Diameter
If the diameter of a circle is 18 units, the radius is half the diameter.
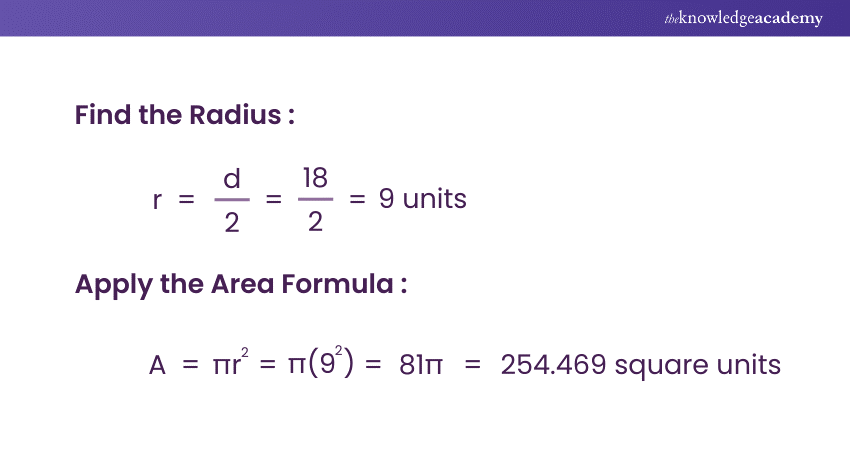
The area is 254.469 square units
Learn how to calculate the Surface Area of a Cylinder and apply the formula with real-world examples. Explore more on our blog now!
Differences Between the Area and Circumference of a Circle
The area and circumference of a circle are related but measure different aspects of the circle. The area measures the amount of space inside the circle, including the Area of Quadrant, and is calculated as πr². It is expressed in square units. The circumference measures the distance around the circle, calculated as 2πr. It is expressed in linear units.
The key differences are:
a) Units: The area is in square units, while the circumference is in linear units.
b) Concept: The area pertains to the space within the circle, whereas the circumference relates to the perimeter around the circle.
c) Calculation: The area depends on the square of the radius, making it sensitive to changes in the radius, while the circumference is directly proportional to the radius.
What is the Perimeter of a Circle?
The perimeter of a circle, also known as the circumference, is the total distance around the circle. It is calculated using the formula:
Circumference = 2πr or πd, where r is the radius and d is the diameter.
What is the Surface Area of a Circle?
The surface area of a circle refers to the area enclosed within its boundary. It is calculated using the formula:
Area = πr², where r is the radius of the circle.
Sharpen your skills. Train to Become a Top Engineer. Join our Engineering Skills Training now!
Conclusion
Congratulations! You've aced the Area of a Circle! Now, along with mastering circles, you can also explore the Area of Square to measure pizzas, fabrics, or any geometric shapes with ease. Remember, practice makes perfect. So next time you see a circle, try calculating its Area of a Circle using the formula you learned. With a little more confidence, you can tackle even more complex shapes!
Take charge of your future! Join our Career Development Course today and unlock the skills and strategies to advance your professional journey.
Frequently Asked Questions
What is the Rule for the Area of a Circle?
The rule for calculating the Area of a Circle is to use the formula A=πr2, where r is the radius of the circle.
What Does the Area of a Circle Depend on?
The Area of a Circle depends on the radius, as it is calculated using the square of the radius, multiplied by the constant π. The radius is the key factor determining the circle's area.
What are the Other Resources and Offers Provided by The Knowledge Academy?
The Knowledge Academy takes global learning to new heights, offering over 3,000 online courses across 490+ locations in 190+ countries. This expansive reach ensures accessibility and convenience for learners worldwide.
Alongside our diverse Online Course Catalogue, encompassing 19 major categories, we go the extra mile by providing a plethora of free educational Online Resources like News updates, Blogs, videos, webinars, and interview questions. Tailoring learning experiences further, professionals can maximise value with customisable Course Bundles of TKA.
What is The Knowledge Pass, and How Does it Work?
The Knowledge Academy’s Knowledge Pass, a prepaid voucher, adds another layer of flexibility, allowing course bookings over a 12-month period. Join us on a journey where education knows no bounds.
What are the Related Courses and Blogs Provided by The Knowledge Academy?
The Knowledge Academy offers various Personal Development Courses, including the Engineering Skills Training, Time Management Training and the Supervisor training. These courses cater to different skill levels, providing comprehensive insights into Best Courses After 12th.
Our Business Skills Blogs cover a range of topics related to Personal Development, offering valuable resources, best practices, and industry insights. Whether you are a beginner or looking to advance your Engineering skills, The Knowledge Academy's diverse courses and informative blogs have got you covered.
Upcoming Business Skills Resources Batches & Dates
Date
Engineering Skills Training
Engineering Skills Training
Fri 16th May 2025
Engineering Skills Training
Fri 18th Jul 2025
Engineering Skills Training
Fri 19th Sep 2025
Engineering Skills Training
Fri 21st Nov 2025
If you wish to make any changes to your course, please
